1st Graders should solve for "x"
- kosukenishimori
- Dec 27, 2022
- 2 min read
From the moment 1st graders learn how to add/subtract and up until 6th grade when students are first exposed to the variable "x," math teaching and problems tend to solely focus on solving for the right side of the = sign.
Textbook problems are designed for students to "practice" their basic addition, subtraction, multiplication, or division problems until they make minimal errors.
If it's a word problem, no skill is required other than to read, seek out, and determine what to do with the given numbers.
Neither is that fun nor develops any kind of critical thinking skill.
As soon as 1st graders learn how to add and subtract single-digit numbers, we need to emphasize mastery of that concept not through repetition, but rather, exploring it from multiple angles.
In an addition problem, this means being able to solve the problem where the unknown "x" is not the total, but rather the beginning or addend quantity.
I am not suggesting that 1st graders use the variable "x." They can supplement the "x" with a ? mark.
Example: Standard Addition Problem
Pam bought 5 pumpkins from the pumpkin patch. Pete bought 4 pumpkins.
How many pumpkins do they have all together?
5 + 4 = ?
Example: Alternative Addition/Subtraction Problem
Pam bought 5 pumpkins from the pumpkin patch. Pete bought some as well and they had a total of 9 pumpkins. How many pumpkins did Pete buy?
5 + ? = 9
Pam bought some pumpkins from the pumpkin patch. Pete bought 4 and they had a total of 9 pumpkins. How many pumpkins did Pam buy?
? + 4 = 9
Obviously the alternative problems are not uncommon and readily available in almost any text or workbook.
I am stating that we need to pro-actively seek more of these types of problems out and have students solve through them.
Straightforward questions elicit this type of response in the student's mind:
"Oh, it asks for the sum, so we probably just add the two numbers together."
Although the pumpkins are also being added in the alternative example, students must critically analyze in their own mind that the solution can be found through subtraction.
The beauty and "fun" in solving math problems should be about students exploring which "tool" they should use from their toolkit.
Below are some suggestions you can implement in your classroom today to get the math juices flowing in your students :)
1. Fewer repetitive computation problems.
2. Choose thought-provoking word problems where the clues are not given (sum, difference, etc.) and students have to analyze the situation.
3. Choose word problems that require students to "work backwards," and the unknown solution is either at the beginning or middle of the equation and not always at the end.
Please let me know how some of these tips work out for the students in your class!
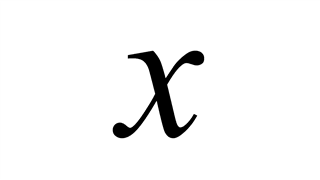













Comments